大气晕

在地球的大气层中,光经常会形成一种只需用肉眼观察天空就可以欣赏到的 奇观,一般来说,大气光现象被称为大气光象,该词源于希腊语“photo”和 “meteora”,分别表示“光”和“在空气中的”[1]。由光与水滴相互作用产生的彩虹和日晕(参阅焦点《壮观的彩虹》和《布罗肯山的光谱》)便是众所周知的例子。冰晶也会产生被称为大气晕的大气光象。
从词源学上讲,“光晕”指的是光环[2],即围绕在太阳、月亮或任何其他光源外部的光圈。大体来说,大气晕是强烈程度不同的光聚集在一起, 在天空中以点、圆或弧的形式出现,主要是由冰晶对光的折射和(或)反射形成。光晕种类繁多,其中一些比较常见的,另一些则十分罕见,甚至只有预测没有观察。有时光晕是彩色的,通过对光晕的观察,可以发现大气中冰晶的特性。
对光晕的最初观测追溯到古希腊和古罗马时期,但直到 17 世纪,一种对于光的科学的方法(综合性、解释性和预测性)随着笛卡尔[3]和惠更斯[4]的研究得以发展。18 和 19 世纪进行了越来越多精确的观察,得益于阿拉戈、巴比内、布拉维、马里奥特、文丘里和杨等物理学家的详细研究。值得一提的是,19 世纪,布拉维斯的毕业论文通篇论述了大气晕[5]。
1. 冰的一些基本性质
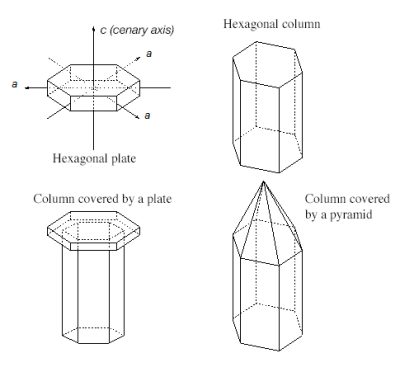
(图中:cenary axis:百分位轴; Hexagonal plate:六角形板;Hexagonal column:六角形 柱; Column covered by a plate:被一个板覆盖的柱子;Column covered by a pyramid:被金 字塔覆盖的柱)
尽管形状多种多样,四种类型的冰晶足以解释大多数的大气晕(图1):六角柱、六角板、柱顶覆小板和所谓的步枪子弹形状(柱顶覆金字塔)。这些晶体有着规则六边形的几何形状,表面或多或少的光滑面构成了光的屈光镜[20],并具有四个对称轴:三个共面轴(a)穿过六边形的顶点并两两形成120°的角,三元轴[6](c)垂直于六边形平面。
大气中最小的晶体尺寸小于50微米,具有布朗(或随机)运动特性,因此没有优先取向。在 50 到 500 微米之间,重力和空气动力摩擦是主导力,因此空 气阻力最小的方向通常是有利的。超过这些尺寸的大晶体围绕水平轴旋转。这些 行为结合晶体的镜面反射[21]和折射[22],解释了光晕的多样性。需要注意的是,空气中同时存在着多种类型和大小的晶体, 因此不同的光晕经常同时出现在天空中,形成所谓的光晕系统。
此外,冰有两个折射率,一个沿a轴,另一个沿c轴,二者十分接近,从紫色略减为红色;平均值约1.31。这种变化解释了为什么由折射形成的光晕在原则上是彩虹色的。而彩虹色中红色、橙色和黄色的亮度比绿色、蓝色更加强烈,当然原因之一在于天空的背景是蓝色。
尽管光晕数量众多,外观各异,但是可以通过以下几种方式进行分类:观测频 率、形状、折射和反射次数(内部或外部)、晶体取向程度以及大小。 上图是按形状进行分类。
2. 由随机和等概率方向小晶体产生的光晕
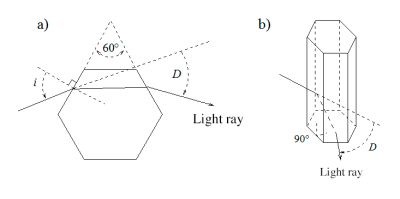
22°光晕—这是最常见的光晕(请参阅文章的封面图片)。它是一个圆环,以太阳为中心,角半径非常接近22°,角宽度[23]约为1到2°。内缘非常清晰,有时呈红色;外部却不那么简单,有时蓝色会拉向白色(封面图片)。所有这些特性均可用由六边形晶体的侧面变化所引起的光线偏差来解释,六边形晶体构成顶角60°的棱镜[7](图2a)。该偏差在22°附近达到绝对最小值,光聚集于此(参阅焦点《棱镜引起的光偏》)。非常多的棱镜呈现0到360°之间的等概率取向,因此光晕在天空呈现圆形[8]。
46°光晕—虽然与22°光晕类似,但这个更宽的光晕不太频繁且更难观察,一方面因为光被重新分布在天空的大部分区域,另一方面因为不利的晶体高宽比。该光晕涉及形成直角棱镜的正交相邻面(图 2b);与这种棱镜对应的最小偏差为46° (参阅焦点《棱镜引起的光偏》)。卡文迪什最早对此提出了解释[9]。
不寻常的光晕—其他更罕见的光晕来自不同角度的棱镜。理论上讲, 所有0到99.5°之间的值都有可能,角半径在 0 到 80°之间的光晕都可观察(参阅焦点《棱镜引起的光偏》)。实际而言,只观察到 4°到 50°之间的光晕:范 · 布仁(8°)、海登(14°)、霍尔(17°)、杜泰尔(24°)、沙纳尔(28°)、 傅叶(32°) 和伯尼(45°) 。这些不寻常的光晕来自形状更复杂的晶体形成的棱镜:金字塔形(图 1)可以解释其中的一些角度;对于沙纳尔光晕来说,来自一种非常罕见的八面体晶体[10]。最后,90°晕被称为赫韦利乌斯晕(1661),几乎观察不到,而且不能用棱镜来解释。
要注意区分光晕和日冕的一个技巧, 日冕与上述的光晕一样, 也是圆形大气光象: 当观察者从中心移开时,光晕从红色变为紫色;日冕则相反,因为是由光的衍射引起的。
3. 中等尺寸晶体产生的光晕

22°的幻日。
幻日—幻日[11]或称假太阳,是一个与太阳高度相同且有一定角度距离的光点。有时成对地出现在太阳的对称的两侧[12] [13]。
幻日常常出现在22°光晕附近。封面图片上伴随22°光晕,两个明亮的光点对称地分布在太阳两侧。它的形成离不开 60°的棱镜,但棱柱的边缘是垂直的, 就像三级轴一样。方向是决定性的,因为入射光线相对于晶体的主要水平截面是倾斜的,穿过晶体后,集中在大于 22°的方位角距离(布拉维定律)[14]。这就是为什么在远离太阳时幻日在水平方向上传播(图3);只有当太阳在地平线上很低,幻日才会被附着在 22°的光晕上。此外,幻日是有颜色的,红色时比蓝色更靠近光晕(图 4)。

照片是作者于 2015 年 11 月 9 日, 7 点 30 分(焦距 78 毫米, 曝光 1/500)在维伦纽夫拍
摄。
更为罕见的一些幻日距太阳的方位角绝对值超过90°。最著名的例子是±120°°;后者是无色的。它们是在晶体垂直的表面上偶数次内反射和在平行表面 上的折射产生的(图 5)。1951年由瑞典气象学家利里奎斯特[15]在南极洲发现了介于 150°到 160°之间的幻日。它们有时被称为偏阳星,因为它们位于面向太 阳的点(方位角 180°) 的两侧, 称为反日点(“反”的意思是“相反”)。在这一点上,一个非常罕见的,未着色的光晕可能出现,其形成过程尚不确定。
出现在水平线上的幻日和光源被称为almicantarat(法语,地平纬圈);后者具体化为称作幻日圆[16]的完整或部分的白色光圈,如果光在没有特殊方位的垂直面上反射。幻日有时垂直传播,形成所谓的幻日弧;产生于垂直方向上可能存在的小波动。
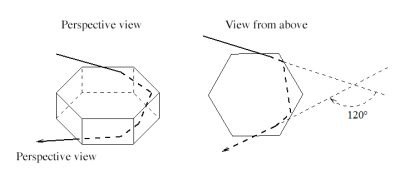
(图中:Perspective view:透视图; View from above:从上面看)
光柱—当太阳刚好位于地平线上方时,就会出现这些狭窄的垂直带。呈现出与光源相同的颜色,由相对于垂直方向略微倾斜的三级轴晶体底部掠入射的反射形成。当方向完美时,光柱薄而明亮;任何对垂直排列的偏离都会使光柱变宽并降低亮度。从飞机上可以看到太阳下的光柱。
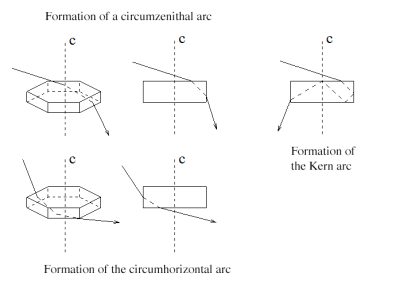
(图中:Formation of acircumzenithalarc:环天顶弧的形成;Formation of acircumhorizontal arc:环地平弧的形成;Formation of the Kern arc:克恩弧的形成)
环天顶弧和环地平弧—朝向天顶或地平线时,这些互补的弧是明亮的,从红色到蓝色呈彩虹色。它们由具有垂直 c 轴和水平 c 轴的直角棱镜的边缘晶体形成,可伴随 46°光晕一起出现。如果光从上表面进入晶体并从垂直表面出射,则弧是环天顶弧(或布拉维弧,图 6);如果光从垂直表面进入并从下表面出射,则弧是环地平弧(图 6)。前者(图 7)部分围绕天顶,并且仅在太阳高度角小于 32°时出现。若大于 32°,则反射全部发生在晶体的内垂直侧面。在32°, 半径出现掠食, 所以是垂直的,环天顶弧成为一个亮点出现在天顶。太阳高度为 22°时,弧线最亮;出现时会于 46°处接触光晕。环地平弧部分围绕地平线,因此可能受景观掩盖,也会于 46°处接触光晕:仅当太阳高度角大于 58° 时环地平弧才会存在,并且在太阳高度角为68°时发光最亮[17]。

在环天顶弧的前面, 我们有时会看到一条以天顶为中心的完整的圆弧:克恩弧。形成于光线穿过相对的垂直面之前的内部反射(图 7)。
帕利弧—由水平c轴晶体形成,具有成对的相对面,也是水平的。它们处在22°光晕的上方和下方,凹面朝向光晕;成对出现,但却混成一团。帕利弧以英国海军上将威廉 · 爱德华 · 帕利爵士[18]的名字命名,他在19世纪初探索了北极并在游记中描述了许多光晕。
映日—飞机上有时可以看到地平线下的椭圆形光点,与太阳对称;那就是日下晕,由水平冰晶面反射阳光而形成的。如果晶体排列得很整齐,在太阳下就是圆形的。这个光环偶尔会被称作布林结环的光环所覆盖。
4. 大晶体产生的光晕
大晶体旋转时,会形成罕见而复杂的光晕,外观在很大程度上取决于太阳的高度。这些弧线,横向正切于光晕,有些呈 22° , 而有些呈 46°。由于通过折射形成的,所以原则上呈现彩虹色。
光环外切到22°光晕—当太阳接近地平线时,在 22°光晕的上方和下方,形成两条与之相切的弧线。当太阳从天空中升起时,两条拱形线会随着相互靠近而变长;当太阳高度角达到 30°时,它们环绕在 22°光晕处。随着太阳继续上升, 这个光环缩小,变得对称, 并最终在太阳高度为 70°处与光晕合并;弧线由 60°棱柱和水平 c 轴围绕旋转而形成。
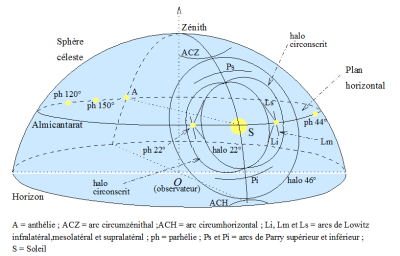
(图中:Almicantarat:Sphèrecéleste:天球; Zénith:天顶;halo circonscrit:Plan
horizontal:水平面;Horizon:地平线;observateur:观察员;A=anthélie:幻日;ACZ=arc circumzénithal:环天顶弧;ACH=arccircumhorizontal:环地平弧;Li, Lm et Ls=arcs de
Lowitz infralatéral,mesolatéralet supralatéral:下, 中外侧和上外侧洛维兹弧;ph=parhélie: 幻日; Ps et Pi=arcs de Parry supérieuretinférieur:上下帕利弧; S=Soleil:太阳)
洛维兹侧弧—三条弧线在22°处穿过幻日点:上侧弧和下侧弧分别位于太阳上方和下方;中外侧弧垂直穿过幻日。1790 年,德俄化学家托拜厄斯 洛 维兹[19]在圣彼得堡首次观察到洛维兹。这些弧是由绕 a 轴旋转的板状冰晶面组成的 60°角棱镜形成的。
46°处的晕的切线和侧弧—棱镜为90°。切线弧通过很多数值模拟得到预测;侧弧(上侧和下侧)拍到的不多,十分少见。
5. 影响参数
到目前为止,我们已经考虑了表面光滑的规则几何形状的晶体,晶体由一个点光源照亮,可以运用著名的几何光学定律加以分析。如果现实真的如此简单,应该可以看到更多的光晕。但事实上,云并不总是均质的晶体介质,通常由于具有散射光的粗糙表面、不规则的几何形状、对称缺陷和内部杂质(气溶胶、气泡) 干扰甚至破坏光晕的形成。
冰晶密度也很重要:光路通过几个晶体的过程中可能连续发生改变;形成多重散射,例如造成+/-44°的幻日现象(光束在±22°处被两个冰晶偏转两次)。此外,光源(太阳、月亮) 较宽;每个点源都有自己的光圈系统。后者重叠并由此产生 的光晕看起来更宽、更不清晰,并且颜色变淡。最后,对于小晶体来说,经常发生的衍射也会使光晕变得更宽、更加不清晰并且颜色更淡。因此,对光晕的观察可以揭示关于云的均匀程度及其组成晶体的特征的大量信息。
光晕研究涉及到大气光学、气象学和冰晶学等各方面。它的种类及其性质取 决于冰晶的几何形状和它们在空间中的方向角度。其中一些光晕原因仍然不确定, 甚至无法解释;比如,椭圆光晕和位于太阳和 22°光晕上部之间的莫拉宁“V”弧。光晕为我们揭示了哪些关于冰晶的信息?反过来,给定的冰晶几何形状可以形成哪些光晕?最后,其他行星会看到光晕吗?数值模拟和观测是回答这些问题的关键。希望这篇关于地球光晕特征的文章会激发读者对这些美丽的大气光学现象 的好奇心,受到鼓舞去仰望天空。愿我们每个人都是细心的观察者,拥有敏锐的头脑,因为我们都是光晕的潜在探索者!
参考资料及说明
封面图片:22°虹彩光晕 (内边缘清晰, 红色,外边缘更弥散,蓝色) 。图片来源:埃尔 维 ·赫尔宾(大气光学实验室(LOA), 里尔)。
[1] A. Rey:法语历史词典。埃德 ·勒罗伯特(2012),4200 页(本文所有词源注释均出自该 词典。)
[2] “halo”一词来源于希腊语“halôs”,指的是小麦被打磨以将谷物从捆中分离出来的区域(圆 盘), 由此延伸出一个圆形的表面, 然后是一个光环。
[3] 笛卡尔:屈光度。流星。在“方法的话语, 正确地引导理性, 在科学中寻求真理” 中, 1637, Ed. de Ch. Angot, Paris, 1668。
[4] 克里斯蒂安惠更斯的完整作品:冠和假日的处理。1662 年或 1663 年。荷兰科学学会,第 17 卷。
[5] 布拉维斯, 1847:晕回忆录和伴随它们的光学现象。皇家理工学院杂志,卡希尔 39 号, XVIII,巴黎。该文章并没有真正过时, 它在观察和理论解释方面提供了非常详细的描述, 并包含了大量的历史参考文献。
[6] 这个轴被称为六元轴,因为晶体以相同的结构绕这个轴 360°旋转六次。
[7] 这个棱镜的解释是由于玛丽奥特:玛丽奥特的作品,《沙发上的肖像》,1686 年。
[8] 这是居里原理的一个例证,根据该原理,结果(光晕几何)至少具有原因的对称性(晶 体取向的等概率)。
[9] 根据 T. Young,这是一门关于自然哲学和机械艺术的讲座课程。1807,卷 II,308 页(“卡 文迪什先生很可能会这么说 ……” )。
[10] 惠利, 1981,沙纳尔光晕:大气中含冰的证据. Science, 211, 389-390
[11] 希腊语“para”和“helio” 的意思是“近”和“太阳”。
[12] 参见历史文本:BravaisA.,1845:注意位于与太阳相同高度的幻日。皇家理工学院杂志,卡希尔 30 号,XVIII,巴黎。
[13] 如果光源是月亮,我们就会说 paraselene (Selene 是希腊神话中的满月女神)。
[14] 布拉维定律涉及入射角度倾斜的光束的折射,即相对于晶体主截面的平面形成非零角 θ。 有两条法则:(1)入射半径和出射半径都是倾斜的(倾角 θ 不变);(2)投射在主截面平 面上的入射半径按照斯涅尔- 笛卡儿定律发生偏转,仿佛它遇到了折射率为[1 + (n²- 1)/ cos²θ]1/2 的棱镜
[15] 利里奎斯特, 光晕现象和冰晶体,在挪威-英国-瑞典南极考察队,1949-52,科学结果, 第二卷,第 2A 部分, 奥斯陆,1956.
[16] 这个名字是因为巴比内(巴比内 1837:气象学备忘录,科学院,638-648)。
[17] 58°和 68°与 32°和 22°互补: 90°- 32° = 58° , 90°- 22° = 68°。
[18] 帕里,《发现从大西洋到太平洋的西北航行杂志》; 1819- 1820 年, 在国王陛下的赫克拉和格里珀船…… 附录包含科学和其他观察的内容……伦敦(1821)。
[19] 洛维兹,1794,关于 1790 年 6 月 18 日在圣彼得堡观测到的一颗非凡的流星的描述。帝国石油新学院, 8384-388。
[20] 两种具有不同光学性质的介质之间的分离表面。
[21] 根据笛卡尔定律,入射光束的反射只在一个方向上进行。如果光束向几个方向反射,则称该反射为漫反射。
[22] 光通过折光面时的偏差。
[23] 角距离(半径、宽度、尺寸等) 是一个圆的中心点(或角度的顶点) 和观察者所在点之间的夹角。我们也说到了明显的距离。
环境百科全书由环境和能源百科全书协会出版 (www.a3e.fr),该协会与格勒诺布尔阿尔卑斯大学和格勒诺布尔INP有合同关系,并由法国科学院赞助。
引用这篇文章: PUJOL Olivier (2024年3月4日), 大气晕, 环境百科全书,咨询于 2024年4月20日 [在线ISSN 2555-0950]网址: https://www.encyclopedie-environnement.org/zh/air-zh/atmospheric-halos/.
环境百科全书中的文章是根据知识共享BY-NC-SA许可条款提供的,该许可授权复制的条件是:引用来源,不作商业使用,共享相同的初始条件,并且在每次重复使用或分发时复制知识共享BY-NC-SA许可声明。